|
Jonathan Smith:
New developments with octonions and sedenions.
The real division algebras
reals R, complex numbers C, quaternions H, Cayley numbers (or octonions) K,
of successive dimensions 1, 2, 4, 8, have progressively deteriorating properties:
- C is no longer ordered;
- H is no longer commutative;
- K is no longer associative.
On the other hand, they all have the property that the Euclidean norm | x | of a vector
x = ( x1, ..., xn )
given by
| ( x1, ..., xn ) | 2 = x12 + ... + xn2
is multiplicative, in the sense that
| x y | = | x | . | y | .
The associative algebras may be described easily as algebras of real matrices, the complex numbers consisting of matrices of the form
and the quaternions consisting of matrices of the form
| [ | t | x | y | z | ] |
| [ | - x | t | - z | y | ] |
| [ | - y | z | t | - x | ] |
| [ | - z | - y | x | t | ] |
with real x, y, z, t. The construction of the Cayley numbers is less immediate, since instead of associativity they only satisfy the weaker Moufang identity
x (( y z ) x ) = ( x y )( z x ) .
[ Note that the Moufang identity specializes to the non-trivial flexible identity
x ( y x ) = ( x y ) x
on setting z = 1 . ]
There are three approaches to constructing the Cayley numbers. The first two are iterative processes: given a multiplication and a conjugation on a real vector space A of dimension 2r, they produce a multiplication and a conjugation on the real vector space A + Ai of dimension 2r + 1. For typographical convenience, the conjugate of a vector x in A will be written as x '. In both processes, the new conjugation is given as
( x + y i ) ' = x ' - y i .
The Cayley-Dickson Process defines the multiplication using the formula
( x + y i ) . ( u + v i ) = ( x u - v' y ) + ( v x + y u' ) i .
- From the reals, it produces the complex numbers.
- From the complex numbers, it produces the quaternions.
- From the quaternions, it produces the Cayley numbers.
After that, it continues to produce algebras satisfying both distributive laws, but the Euclidean norms are no longer multiplicative, and indeed the algebras start to have zero-divisors. It will be convenient to refer to the 16-dimensional algebra produced in this way from the Cayley numbers as the Cayley-Dickson sedenions.
The Conway-Smith Process defines the multiplication using the formula
( x + y i ) . ( u + v i ) =
( x u - ( y ' v ) ' ) +
( y u ' + y ( x ' ( y - 1 v ) ' ) ' ) i ,
in which the latter coefficient of i is interpreted as ( x ' v ' ) ' if y is zero.
- From the reals, it produces the complex numbers.
- From the complex numbers, it produces the quaternions.
- From the quaternions, it produces the Cayley numbers.
After that, it continues to produce "semialgebras" satisfying just one distributive law, but with multiplicative Euclidean norm. It will be convenient to refer to the 16-dimensional algebra produced in this way from the Cayley numbers as the Conway-Smith sedenions.
The third approach to constructing the Cayley numbers is more combinatorial. Begin with the complex numbers as the real space R e0 + R e1, with the product given by making e0 the identity element, defining
e1 . e1 e { e1 , - e1 } ,
and choosing the sign of ej . ek from the matrix:
This sign matrix S or SC of the complex numbers with respect to the basis
{ e0 , e1 }
is a normalized Hadamard matrix: its first column and first row consist just of ones, its other entries are all either 1 or - 1, and it satisfies the condition
S S T = n I ,
n being the size 2 of S.
Now take the quaternions as H = R e00 + R e01 + R e10 + R e11, where e00 is the identity element, e01 is the matrix
| [ | 0 | 1 | 0 | 0 | ] |
| [ | - 1 | 0 | 0 | 0 | ] |
| [ | 0 | 0 | 0 | - 1 | ] |
| [ | 0 | 0 | 1 | 0 | ] |
e10 is the matrix
| [ | 0 | 0 | 1 | 0 | ] |
| [ | 0 | 0 | 0 | 1 | ] |
| [ | - 1 | 0 | 0 | 0 | ] |
| [ | 0 | - 1 | 0 | 0 | ] |
and e11 is the matrix
| [ | 0 | 0 | 0 | 1 | ] |
| [ | 0 | 0 | - 1 | 0 | ] |
| [ | 0 | 1 | 0 | 0 | ] |
| [ | - 1 | 0 | 0 | 0 | ] |
The sign of ej . ek is chosen according to the matrix SH , namely
| [ | 1 | 1 | 1 | 1 | ] |
| [ | 1 | - 1 | 1 | - 1 | ] |
| [ | 1 | - 1 | - 1 | 1 | ] |
| [ | 1 | 1 | - 1 | - 1 | ] |
which is again a normalized Hadamard matrix. (Think of the rows and columns being numbered from 0 to 3 in binary notation.) For non-identity ej , one has
ej2 = - 1 .
For distinct non-identity ej , ek , one has
ej . ek e { el , - el } ,
where { j , k , l } is the triple { 01, 10, 11 }.
The combinatorial construction of the Cayley numbers continues this process, using a real basis consisting of the identity element e000 and the remaining seven elements e001 , ... , e111 with suffices running through the binary representations of 1 , ... , 7. For non-identity ej , one again has
ej2 = - 1 .
For distinct non-identity ej , ek , one has
ej . ek e { el , - el } ,
where { j , k , l } is a line from the Fano plane
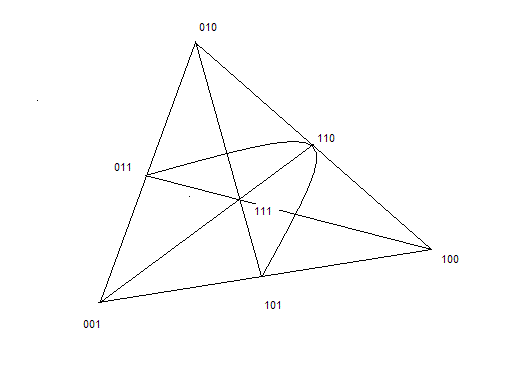
(including the "curved line" with points 011, 110, 101).
The sign matrix SK is an 8 x 8 normalized Hadamard matrix, namely
| [ | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | ] |
| [ | 1 | - 1 | 1 | - 1 | 1 | - 1 | - 1 | 1 | ] |
| [ | 1 | - 1 | - 1 | 1 | 1 | 1 | - 1 | - 1 | ] |
| [ | 1 | 1 | - 1 | - 1 | 1 | - 1 | 1 | - 1 | ] |
| [ | 1 | - 1 | - 1 | - 1 | - 1 | 1 | 1 | 1 | ] |
| [ | 1 | 1 | - 1 | 1 | - 1 | - 1 | - 1 | 1 | ] |
| [ | 1 | 1 | 1 | - 1 | - 1 | 1 | - 1 | - 1 | ] |
| [ | 1 | - 1 | 1 | 1 | - 1 | - 1 | 1 | - 1 | ] |
There are a number of currently active research projects involving the octonions, sedenions, and related algebras:
- J.D. Phillips and P. Vojtechovsky have been classifying Moufang-like identities connecting words of length 4 in three variables. As part of their classification, they have recovered identities originally studied by Fenyves, essentially saying that squares of elements associate with any pair of elements. Cawagas has discovered 16-element subloops of the Cayley-Dickson sedenions that provide the first known non-trivial natural models of Fenyves' identities. The breakdown of the Moufang law for these loops appears related to the existence of zero-divisors.
- The integers in the octonions form a copy of the E8 lattice. For example, there are 240 integral octonions of norm 1. They form a subloop of the octonions, a double cover of the smallest non-associative simple Moufang loop whose character table was determined by S.Y. Song in his Ph.D. thesis. Derek Smith is investigating the further structure of octonionic integers. So far, little work has been done to investigate the integers of other algebras.
- There is a continuing search for subloops of these algebras, with the eventual goal of a full classification including an analysis of the identities that they satisfy. The classical inspiration for this project is the classification of subgroups of the quaternions, which subsumes the classification of the Platonic solids and related graph-theoretical classifications.
- B. Kivunge is investigating the combinatorial structure of the higher-dimensional semialgebras, relating them to higher-dimensional projective geometries over GF(2).
- M. Bremner and I.R. Hentzel have been investigating identities (e.g. the flexible law) satisfied by the full Cayley-Dickson algebras.
- These algebras continue to be of interest for their potential relevance to physics, including spacetime modeling and connections with string theory and GUTs. The quaternions provide good models for spinors, and are also now used to drive graphics in video games and similar applications.
Back to the seminar listing
|
|